Given that xyz= 1, You will get minimum value only when x=y=z So in this given case, you will get minimum value of x^2y^2z^2 when x=y=z= 1/3 So minimum valueTo ask any doubt in Math download Doubtnut https//googl/s0kUoeQuestion If 2^x = 3^y =12^z show that 1/z= 1/y2/xXyz=2, 2x2y2z=4, xy=2 Extended Keyboard;

How To Solve If X Y Z 0 Find The Value Of Y Z 2 Yz Z X 2 Zx X Y 2 Xy Mathematics Topperlearning Com 7bcu50oo
F(x y z)=x^2 y^2 z^2 x y z=12
F(x y z)=x^2 y^2 z^2 x y z=12-The Roman surface or Steiner surface is a selfintersecting mapping of the real projective plane into threedimensional space, with an unusually high degree of symmetry This mapping is not an immersion of the projective plane;Show that the intersections of this surface with planes perpendicular to the xand yaxes are hyperbolas Hint Set either y = c or x = c for some constant c The other type is the hyperboloid of two sheets, and it is illustrated by the graph of x 2 y 2 z 2 = 1, shown below
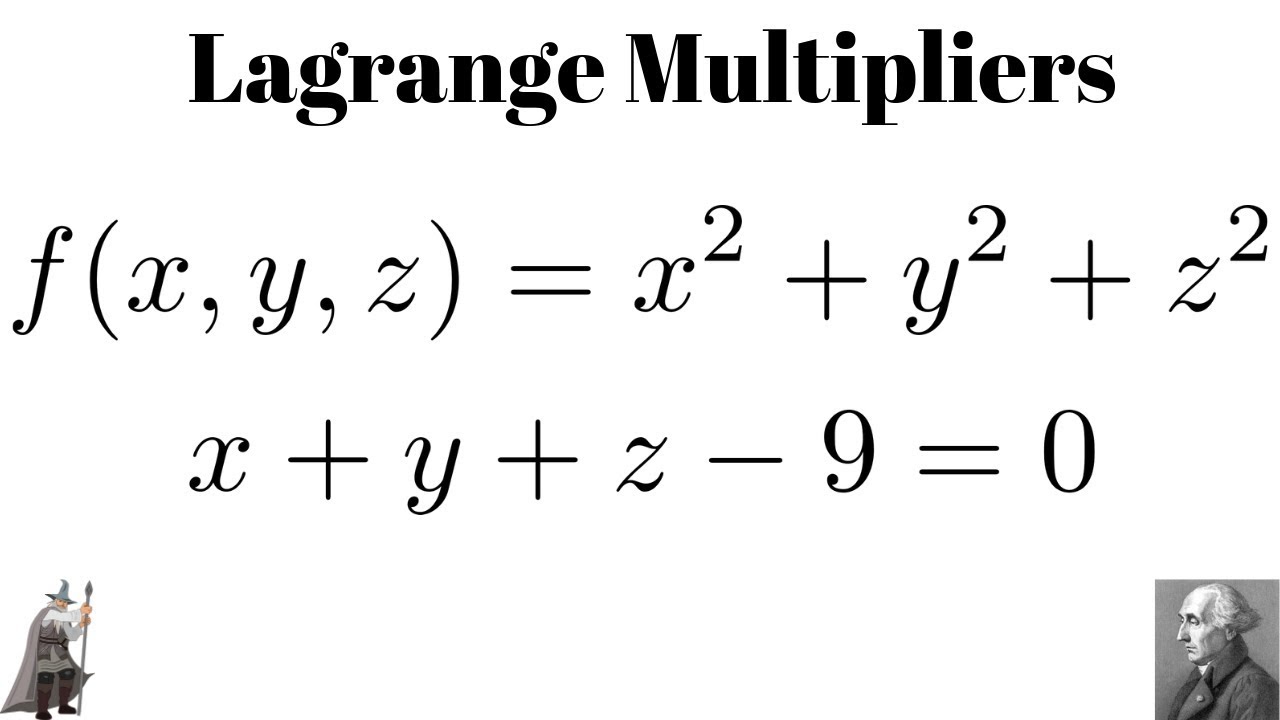


Lagrange Multipliers Minimum Of F X Y Z X 2 Y 2 Z 2 Subject To X Y Z 9 0 Youtube
S is defined as a sphere · $(xyz)(x^2y^2z^2)=x^2y^2z^2x^2yx^2zxy^2y^2zxz^2yz^2=2$ buradan da $3(x^2y^2z^2x^2yx^2zxy^2y^2zxz^2yz^2)=6$ denklemi elde edilir Denklemleri taraf tarafa çıkarırsakLetf (x,y,z) = x^2y^2z^2 Calculate the gradient of f Calculate ∫_C (F dr ) where F (x,y,z)= (x,y,z) and C is the curve parametrized by r (t)= (3cos^3 (t),
1217 · how can i draw graph of z^2=x^2y^2 on matlab Learn more about surface MATLAB C/C Graphics LibraryX y z = 16 x z = 12 y = 2 Log On Algebra Matrices, determinant, Cramer rule Section Solvers Solvers Lessons Lessons Answers archive Answers Click here to see ALL problems on Matricesanddetermiminant;However, the figure resulting from removing six singular points is one Its name arises because it was discovered by Jakob Steiner when he was in Rome in 1844 The simplest construction is as the image of a sphere centered at the origin under the map f
Free math problem solver answers your algebra, geometry, trigonometry, calculus, and statistics homework questions with stepbystep explanations, just like a math tutor0118 · See below Making m = xy n = yz p = xz we have m^2n^2p^2 = 2(x^2y^2z^2x*yx*zy*z) then x^2y^2z^2x*yx*zy*z = 1/2((xy)^2(yz)^2(xz)^2)This equation is in standard form ax2 bx c = 0 Substitute 1 for a, −x−z for b, and x2 z2 −zx for c in the quadratic formula, 2a−b± b2−4ac y=\frac {\left (xz\right)±\sqrt {\left (xz\right)^ {2}4\left (x^ {2}xzz^ {2}\right)}} {2} y = 2−(−x − z) ± (−x − z)2 − 4(x2 − xz z 2) Square xz



Use Stokes Theorem To Evaluate 5 Curl F Ds F X Y Z X 2z 2i Y 2z 2j Xyzk S Is The Part Of The Paraboloid Z X 2 Y 2 That Lies Inside The Cylinder X 2 Y 2 4



Ex 3 2 12 Given 3 X Y Z W X 6 1 2w 4 X Y Z W 3
Compute answers using Wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals For math, science, nutritionThe idea is that since xyz is cubic, it will be larger than x 2 y 2 z 2 unless one number is much larger than the others But if that's the case, we can always replace the largest number with a smaller one (until x,y,z form an acute triangle)Suppose f(x,y,z)=1/(sqrt(x^2y^2z^2)) and W is the bottom half of a sphere of radius 6 Enter ρ as rho, ϕ as phi, and θ as theta What are the integrated integral and limits of integration using spherical coordinates?



Let Math X 2 Y Z Y 2 Z X Z 2 X Y Math What Is The Value Of Math Frac 1 1 X Frac 1 1 Y Frac 1 1 Z Math Quora
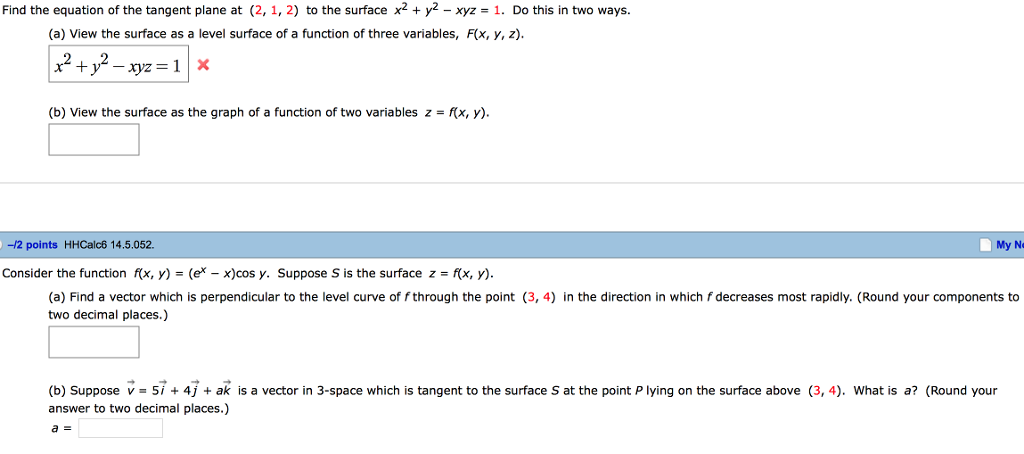


Solved Find The Equation Of The Tangent Plane At 2 1 2 Chegg Com
· 3Dplot of "x^2y^2z^2=1" Learn more about isosurface;En este video encontrarás la solución, paso a paso, del sistema de dos ecuaciones de primer grado, o lineales, con dos incógnitas "1) xyz=12 2) 2xyz=7 En este video encontrarás laIf U = F ( Y − X X Y , Z − X X Z ) , Show that X 2 ∂ U ∂ X Y 2 ∂ U ∂ Y Z 2 ∂ U ∂ Z = 0 University of Mumbai BE Automobile Engineering Semester 1 (FE First Year) Question Papers 141 Important Solutions 525 Question Bank Solutions 527 Concept Notes 24 Time Tables 23 Syllabus



Solved Finding An Equation Of A Tangent Plane In Exercise Chegg Com



Let Math X 2 Y Z Y 2 Z X Z 2 X Y Math What Is The Value Of Math Frac 1 1 X Frac 1 1 Y Frac 1 1 Z Math Quora
2x0 d dx z2 2 x 0 d d x z 2 Since z2 z 2 is constant with respect to x x, the derivative of z2 z 2 with respect to x x is 0 0 2x0 0 2 x 0 0 Combine terms Tap for more steps Add 2 x 2 x and 0 0 2 x 0 2 x 0 Add 2 x 2 x and 0 0 2 x 2 x · Verify Stokes theorem for F =(y^2 x^2 x^2)i (z^2 x^2 y^2)j (x^2 y^2 z^2)k over the portion of the surface x^2 y^2 2ax az = 0 While evaluating the integral we get hard to evaluate integrals What can we do to simplify this?Step by step solution of a set of 2, 3 or 4 Linear Equations using the Substitution Method 2xy=2;xyz=2;5x2y5z=6 Tiger Algebra Solver



13 7 Extreme Values And Saddle Points Mathematics Libretexts



X Y Z Belong To R X Y Z 4 X 2 Y 2 Z 2 6 Find Maximum Possible Value Of Z Mathematics Topperlearning Com 2j1z7sww
· Once we find these, we can construct any symmetric polynomial in x, y and z We are given x y z, so we just need to derive the other two Note that 2(xy yz zx) = (x y z)2 −(x2 y2 z2) = − 1 So xy yz zx = − 1 2 Note that 6xyz = (x y z)3 −3(x y z)(x2 y2 z2) 2(x3 y3 z3) = 1 SoAnd then now we are given the constraints Um, X plus two I was three z equal six and expose three way place nine z is equal to nine So we are gonna be using equation to for this problem that is given in the book for those problems that have two constraints and that says we want to solve the greeting of f is equal to land The times the grating of G one plus mu times the Grady int g tooMinimize f(x, y, z) = x^2 y^2 z^2 subject to 4x^2 2y^2 z^2 = 4 Maximum Valua At (,,) (1 pt) Find the coordinates of the point (x, y, z) on the plane z = 2 x 2 y 3 which is closest to the origin x = 2 y = z =


If A X B B Y C C Z A What Is Xyz Quora



Applications Of Partial Derivatives Calculus 3


